Ptolemy’s geocentric model

It is not rare that people make mistakes in maths; the frustration caused by those mistakes sometimes torments people a lot. Here is one fact that might relieve the frustration: even the smartest and best mathematicians make huge mistakes, and that does not damage their fame. In fact, Ptolemy, a Greek mathematician who lived in the 2^nd century, is famous for his geocentric model which consists of tons of mistakes.

Praise across the world

The influence of Ptolemy on astronomy is so great that his work is widely regarded as the summit of ancient astronomy. His work Mathematical Syntax was translated by Arabs and renamed as Almagest, meaning “the greatest”. Owen Jay Gingerich, professor at Harvard University, praises Ptolemy’s Syntaxis with the words “the apex of ancient astronomy.” G. E. R. Lloyd, professor at the University of Cambridge, writes in The Cambridge Ancient History: “he [Ptolemy] produced models … must rank as one of the most outstanding achievements of ancient science.” And Reviel Netz, professor at Stanford University, comments: “his [Ptolemy’s] project canonized soon after his death. Eventually, he would be one of the pillars of the scientific canon emerging in Late Antiquity” plus he uses “Culmination: Ptolemy” as the title of a subchapter.

You may wonder why these experts praise Ptolemy’s geocentric model which has long since been dumped into the trash bin of discarded theories? The answer is that Ptolemy’s Almagest, more than any other book, convinced people that the seemingly complex phenomena of the heavens could be represented by a simple underlying mathematical description. Although there are evidences showing that positions of celestial bodies and the times of celestial events could already be predicted by Babylonians and Chinese centuries before Ptolemy, he firstly use maths to give out a clear ontological reference, before him, maths is only limited to the calculation for the prediction of certain astronomical phenomena rather than models that correspond. Harnessing untamed nature into a mathematical theory is not a simple task, because observational data are not quite as tidy as the abstractions of pure points and lines, but Ptolemy eventually achieved that.

Show this chapter

Models and Mistakes

A ink portrait of Claudius Ptolemäus facing to the left. He is wearing stripped formal outfit. — Claudius Ptolemäus.
Image credit: Theodor de Bry, public domain, via Wikimedia Commons.

In Book I of Almagest, Ptolemy introduced his model: “the heaven is spherical in shape, and moves as a sphere; the earth too is sensibly spherical in shape, when taken as a whole; in position it lies in the middle of the heavens very much like its centre; in size and distance it has the ratio of a point to the sphere of the fixed stars; and it has no motion from place to place.” Despite some inconsequential problem, such as the centre of universe, there are two important mistakes that he made. Firstly, the rotation and revolution of Earth are omitted (which is the reason for lots of other mistakes), secondly planets move elliptically around the sun, not circularly around the Earth.

Differing from the public’s stereotypical perception, those mistakes in his geocentric model are not the consequence of religious pressure. It is true that a Stoic philosopher is reported by Plutarch to have thought that the Greeks ought to indict a heliocentric scholar for impiety for putting the ‘hearth of the universe’ [the Earth] in motion. But there is no evidence to show that Ptolemy was influenced by Stoics or Christianity which was only legalized in the Roman empire about 15 decades later. Conversely, those mistakes are a consequence of logic: Ptolemy fully evaluates his assumptions with pros and cons before he made it the foundation of model.

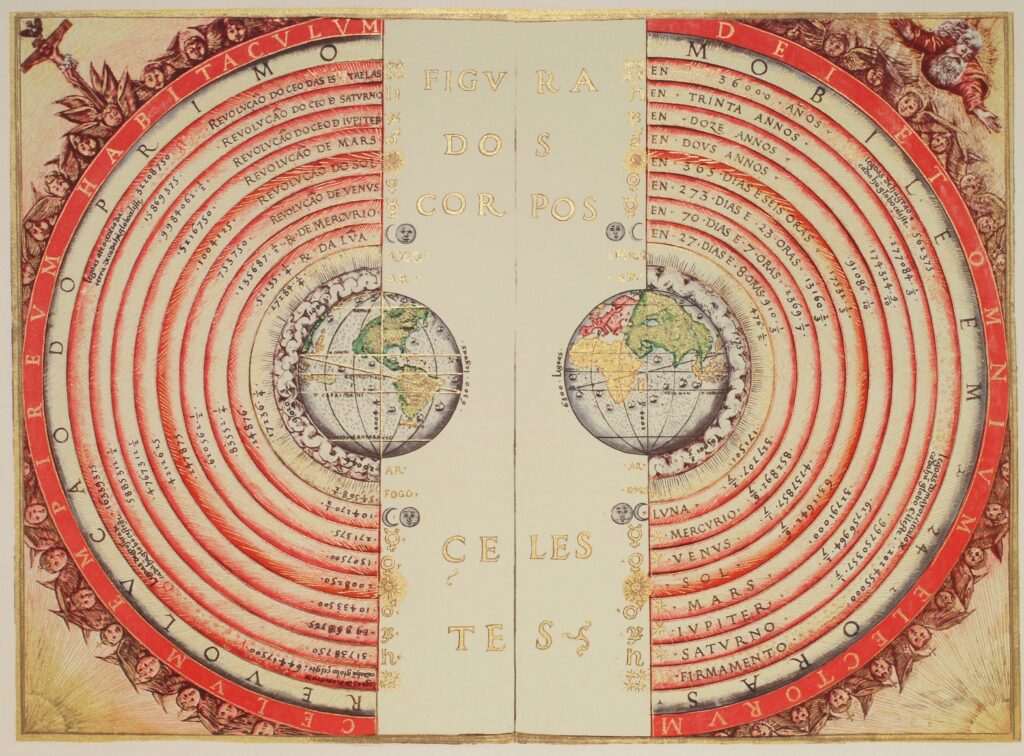
A figure of two circles of the globe with vertical semi circles label of the planets. — Figure of the Heavenly Bodies, the Ptolemaic geocentric model of the universe.
Image credit: Bartolomeu Velho, public domain, via Wikimedia Commons.

Four centuries before Ptolemy, Aristotle had already recorded the existence of gravity in his On the Heavens: There is a unique movement of most matters, excluding air, clouds etc., toward the centre of our planet. So naturally, he stated:

“The place to which any fragment of earth moves must necessarily be the place to which the whole moves; and in the place to which a thing naturally moves, it [Earth] will naturally rest.”

Ptolemy agreed with Aristotle’s opinion, although he stated that the rotation of Earth could also explain all the movements in the heavens:

“…supposed the heavens to remain motionless, and the earth to revolve from west to east about the same axis [as the heavens], making approximately one revolution each day…”

But he quickly denied that in a long argument with several reasons, one of which I give below:

“Let us concede to them [for the sake of argument] that such an unnatural thing could happen as … the densest and heaviest objects [Earth] have a proper motion of the quick and uniform kind which they suppose. Nevertheless, they would have to admit that the revolving motion of the earth must be the most violent of all motions associated with it, seeing that it makes one revolution in such a short time; the result would be that all objects not actually standing on the earth would appear to have the same motion, opposite to that of the earth: neither clouds nor other flying or thrown objects would ever be seen moving towards the east … Yet we quite plainly see that they do undergo all these kinds of motion …”

Show less

Ptolemy’s argument seems reasonable under the assumption that clouds are unaffected by gravity, which is the reason why clouds are floating in Aristotle’s explanation.

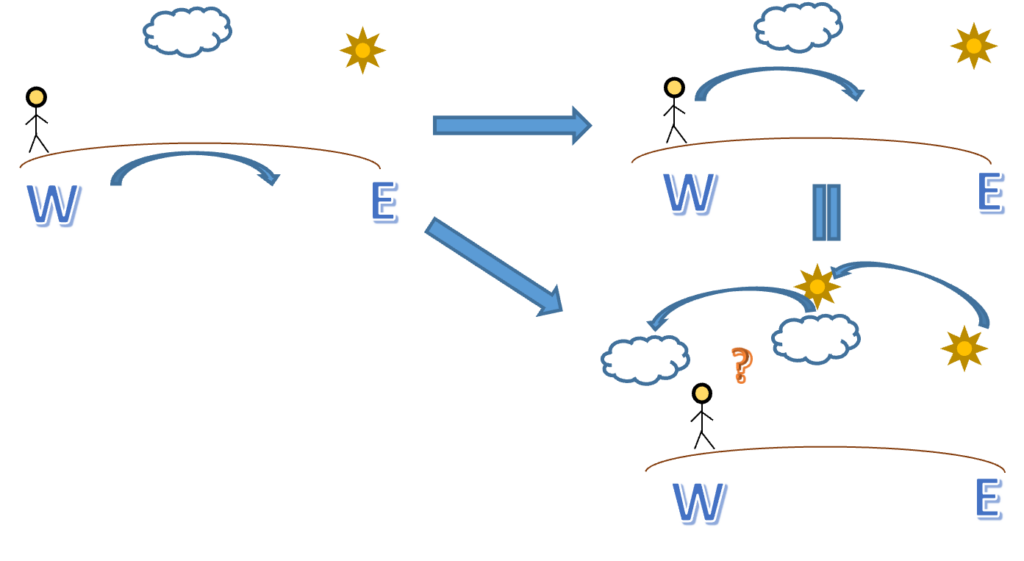
A Diagram of stick figure on a horizontal plane with a sun and a cloud underneath a W with an arrow pointing to E. There is a similar diagram, but the arrow is by the stick figure. There is a third similar diagram with an additional sun and arrows connecting them. — Example graph produced by writer, cloud will move very quickly under Ptolemy’s argument, it at least travels 40,000km a day, so the average speed is at least 1667km/h, while the speed of sound is only 1,235 km/h (Eratosthenes had already calculated out the Earth’s circumference is about 40,000km in about 240 BC)
Image credit: Ammi Tian, CC BY-SA 4.0.

Under the condition that the Earth is not moving, most of the other mistakes come as a consequence. The daily appearance of celestial bodies is then regarded as the revolution around Earth. Ptolemy deduced the wrong conclusion using a wrong assumption:

“To sum up, if one assumes any motion whatever, except spherical, for the heavenly bodies, it necessarily follows that their distances, measured from the earth upwards, must vary, wherever and however one supposes the earth itself to be situated. Hence the sizes and mutual distances of the stars must appear to vary for the same observers during the course of each revolution, since at one time they must be at a greater distance, at another at a lesser. Yet we see that no such variation occurs. For the apparent increase in their sizes at the horizons is caused, not by a decrease in their distances, but by the exhalations of moisture surrounding the earth being interposed between the place from which we observe and the heavenly bodies, just as objects placed in water appear bigger than they are, and the lower they sink, the bigger they appear.”

Show less

End of geocentric model

The majority of mathematicians and astronomers believed in the geocentric model to some extent for 14 centuries until, decades after Copernicus published On the Revolutions of the Heavenly Spheres. Tycho Brahe’s observational data convinced people other planets move elliptically around the sun, which is the second mistake of Ptolemy’s model, and from his data Kepler concluded the law of planet motion. But those laws were just empirical, it couldn’t be a powerful evidence to show earth is not static, until Newton and Hooke developed gravity theory. And the final strike that buried geocentric model is given by Thomas Henderson, his observation confirmed existence of stellar parallax, which is caused by earth motion. Of course we also need thank Galileo Galilei for the invention of telescope, most of the observational used for against geocentric model can’t be collected without telescope.

Although Ptolemy‘s model has been discarded, his work can still inspire people: it is not shameful to make mistakes, just try your best and let the merits outweigh the flaws.

Show this chapter

Show Bibliography

Aristotle, On the heavens II, translated by John Leofric Stocks (Oxford, 1922)

Gingerich, Owen, ‘Islamic Astronomy’, Scientific American, vol. 254, no. 4 (1986)

Heath, Thomas, A history of Greek mathematics (Oxford, 1921) 

Netz, Reviel, A new history of Greek mathematics (Cambridge, 2022)

Ptolemy, Claudius, ‘Almagest’ (Alexandria), translated by G. J. Toomer (New Jersey, 1998)

Walbank, F. W., A. E. Astin, M. W. Frederiksen, and R. M. Ogilvie, eds. The Cambridge Ancient History Volume 7, Part 1: The Hellenistic World, 2nd edition (Cambridge, 1984)

Author
Ammi Tian