Snowflakes and the Art of Mathematical Repetition
Snowflakes, random and delicate, carry within them one of nature’s most enchanting secrets: they are fractals, the geometry of the wild. ‘Fractal’ might sound like a term reserved for mathematicians, but it’s really a simple concept that is as natural as the branches of trees.
The Wonder of Winter’s Lace
Fractals are patterns that repeat at different scales; no matter how close you look, the pattern is always the same. Consider the snowflake, winter’s most delicate fractal. At its core, a snowflake begins with a simple six-sided, symmetrical figure. But as the snowflake falls, swirling through clouds of varying humidity and temperature, its arms reach out, repeating the hexagonal pattern in an ever-growing cascade of miniature splendor.
This repetition on different scales is the hallmark of fractal geometry, and snowflakes are a perfect example. They are not just random ice crystals; they are a symphony of mathematical rules played out in icy miniature. Each snowflake is a frozen snapshot of water vapor obeying the laws of fractal geometry, a testament to the fact that our universe is a place where mathematics and art collide in spectacular fashion.
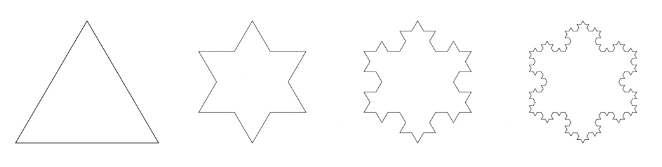
Image credit: Eric Weisstein, fair dealing via Wolfram Mathworld.
The Simple Rules of Complexity
The essence of fractals is the repetition of a straightforward process that gives rise to intricate patterns, each part a miniature echo of the whole. Self-similarity means no matter how much you zoom in on a fractal, you will find the same pattern repeating, from the macroscopic to the microscopic, as can be seen in snowflakes.
But how does such complexity arise from simplicity? The journey of a snowflake begins with a speck—a tiny dust particle in a cloud—which becomes the seed for ice to crystallize upon. As the snowflake starts its descent to Earth, it encounters different pockets of air, each with its own temperature and humidity. These varying atmospheric conditions sculpt the snowflake’s shape as it falls.
The colder and more humid the air, the more intricate the snowflake’s branches become. This process is dynamic and sensitive; even a slight change in conditions can alter the pattern, making each snowflake unique. Yet, despite their individuality, all snowflakes share the same hexagonal symmetry—a rule dictated by the molecular structure of water, which guides their formation.
This interplay of fixed laws and environmental whimsy creates the snowflake’s complexity. It’s a dance of predictability and surprise, where the basic act of freezing water is repeated in a feedback loop, each step influenced by the changing air around it. The result is a fractal—a structure that embodies order and chaos, a pattern that is infinitely complex yet founded on simple, repeating rules.
The Historical Quest to Decode Snowflakes
Wilson A. Bentley, a farmer from the snowy state of Vermont, harboured a fascination for the delicate ice crystals that blanketed his winter world. In the 1880s, armed with a microscope and a bellows camera, Bentley became the first person to photograph snowflakes, calling them “tiny miracles of beauty” and capturing over 5,000 images in his lifetime. His pioneering work unveiled the astonishing diversity and complexity of snowflakes, revealing that no two were exactly alike.
Bentley’s photographs were more than just portraits of frozen water; they were the first steps toward understanding the mathematical tapestry woven into the fabric of each flake. His meticulous records of the conditions under which each snowflake formed began to hint at the underlying rules governing their creation—rules that spoke of the fractal nature of these ice crystals long before the science of fractals was formally developed.

Image credit: fair dealing via Jericho Historical Society.
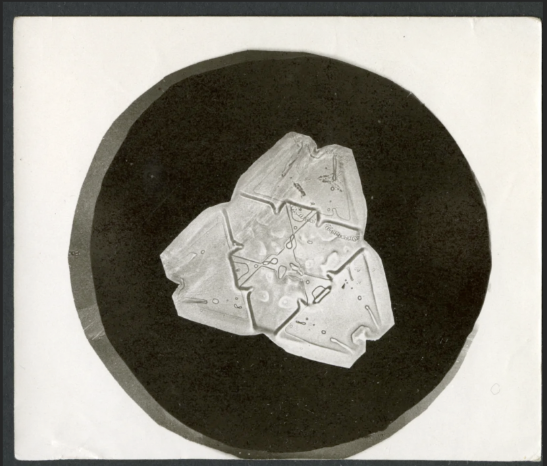
Image credit: fair dealing via Smithsonian Institution Archives.
It was through Bentley’s lens that the world first saw the marriage of math and art in the form of snowflakes. His legacy laid the groundwork for the mathematical exploration of fractals. Researchers would later stand on Bentley’s shoulders, using his detailed observations as a springboard to delve deeper into the mathematical nature of these winter wonders.
Today, Bentley is affectionately remembered as “The Snowflake Man,” a pioneer who not only expanded our understanding of snowflakes but also showed us the profound beauty inherent in the pursuit of knowledge. His work remains a testament to the human desire to understand the natural world, and it continues to inspire scientists and mathematicians to unravel the complex dance of symmetry and chaos that is the fractal geometry of snowflakes.

Image credit: fair dealing via Quanta Magazine.

Image credit: fair dealing via Quanta
Magazine.
Author
Akshitha Vemuru

