Decolonising Mathematics: Indigenous Algorithms from Indonesian Fisheries

When we think of mathematics, we often imagine textbooks, blackboards, and equations rooted in European traditions. But what if the most sophisticated algorithms weren’t written in Latin or Greek, but woven into bamboo traps and net patterns passed down by generations of Indonesian fishers? This article explores how traditional Indonesian fishing communities have used intricate mathematical systems for centuries, and how colonial powers tried to erase them.

Indigenous fishery knowledge is not simply a cultural tradition; it is a repository of ecological mathematics. Long before modern conservationists began modelling fish stocks or mapping spawning cycles, communities across the Indonesian archipelago were applying logic, symmetry, and algorithmic thinking to manage their environments. In the process, they were sustaining biodiversity while also sustaining themselves. These systems were not abstract theories. They were embodied practices, tools designed through observation, trial, and error, and adapted seasonally depending on changing ecological conditions. In this way, Indigenous science operated on principles of responsiveness and sustainability.

**Fishing as Mathematics: The Pencar Net**

In Lombok, Indonesia, the pencar is a circular cast net used in coastal fishing. To the untrained eye, it might look like a simple tool, but hidden in its construction is a mathematical formula: Uₙ = n² + 43n. This quadratic sequence controls the spacing and shape of the net’s mesh. Each added row (called an anakan) follows this sequence to ensure optimal expansion, allowing juvenile fish to escape while catching mature ones. In other words, it’s a built-in sustainability model, crafted without calculators or computers.

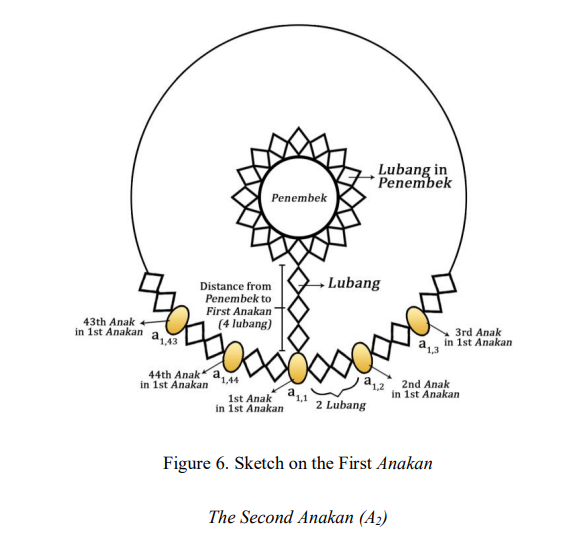
Figure 1. Sketch of the first anakan (widener row) in a traditional pencar net, showing mesh loop patterns based on an arithmetic sequence.
Adapted from Akbar et al., ‘Arithmetic Sequences in Making Traditional Cast Nets in Lombok,’ 2023,

Local fishers adjust their net design based on seasonal tides and fish movement, a dynamic form of applied mathematics shaped by observation and experience. It’s an example of what scholars call ethnomathematics: the study of how different cultures develop their own and unique mathematical systems. These systems are passed down orally and practically, through hands-on training and community practice.

Some net-makers even use local chants or mnemonic patterns to keep track of sequence growth, blending rhythm and mathematics in a unique pedagogical method. The pencar’s form thus encodes environmental adaptation and intergenerational learning, a living proof that science is not only written in books, but also in practice.

**The Geometry of Traps: Bubu Structures**

Further west, in Bintan and other parts of the Riau Archipelago, fishers construct bubu traps, cylindrical or box-shaped bamboo cages. The geometry of a bubu trap is no accident. Mesh spacing follows odd-numbered sequences (15-35-21 holes) that influence water flow and fish movement. These geometric patterns guide fish into the trap while allowing smaller ones to escape. The result? Selective harvesting that supports fish stock regeneration.

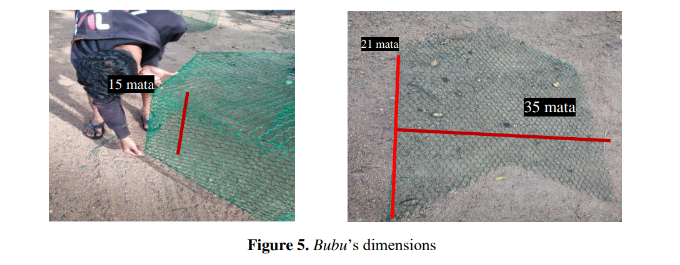
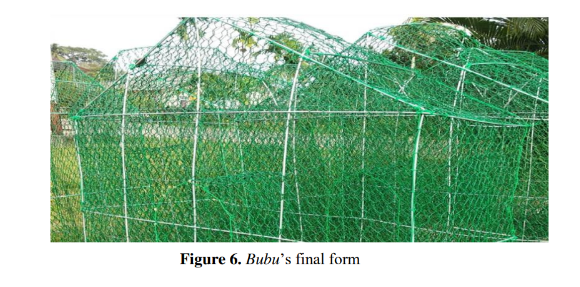
Figure 2. A bubu trap is set on the sand. Its design reflects geometric patterns that promote selective harvesting and natural fish movement. The other picture is here to illustrate its final form. These diagrams illustrate the trap’s modular geometry and sequential mesh design.
Adapted from Febrian, Astuti, and Susanti, ‘Ethnomathematical Study on Indigenous Fish Trap: Example from Kijang, Bintan Regency,’ Jurnal Pendidikan Matematika 17, no. 1 (2023): Figures 5 & 6. https://doi.org/10.22342/jpm.17.1.18787.21-36

The design isn’t just practical, it’s mathematically elegant. Tests by fisheries engineers have shown that these patterns create stable hydrodynamics that reduce pressure on marine ecosystems. These aren’t relics of the past; they’re functioning algorithms, designed through lived experimentation.

The construction of bubu traps often involves circular symmetry and modular bamboo weaving, reflecting knowledge of strength distribution and water resistance. When placed correctly, bubu traps allow water to flow through without disturbing sediment, helping maintain benthic habitats, an ecological function that modern plastic traps often neglect.

Recent ethnographic research has noted how specific proportions within the trap replicate natural cave-like refuges, mimicking marine environments preferred by reef species. These insights show how Indigenous design incorporates both biological knowledge and practical geometry.

Bubu designs also vary across regions, adapting to the depth, current, and type of species being targeted. This regional variation shows that local knowledge is not static but fluid, shaped by micro-environments and centuries of experience. The success of these traps is not only about the shape, but about understanding marine rhythms.

Colonial Disruption: Replacing Knowledge

During Dutch colonisation (1602–1949), these Indigenous systems were viewed as primitive. Laws like the 1935 Fisheries Ordinance replaced community-based regulations (such as the awig-awig laws in Lombok) with European-style quotas and mechanised fishing fleets. Colonial authorities introduced trawlers and purse seines with uniform mesh sizes, ignoring the local knowledge embedded in net variation.

Figure 3. Traditional Indonesian wooden boat with lete sail, photographed in the early 20th century. The craft exemplifies vernacular maritime technology now recognised by UNESCO as intangible cultural heritage. Source: New Mandala, ‘UNESCO Heritage Lists Indonesian Wooden Boat Building,’ New Mandala, July 4, 2017, https://www.newmandala.org/unesco-heritage-lists-indonesian-wooden-boat-building/.

The awig-awig system, rooted in customary law (adat), governed when, where, and how fishing could occur. It was locally enforced and evolved in dialogue with environmental shifts. By stripping these laws of legal authority, the colonial regime undermined the ability of local communities to self-regulate sustainably.

This wasn’t just a change in tools; it was a change in worldview. The Dutch dismissed adaptive Indigenous methods as unscientific, while imposing rigid models that failed to account for ecosystem diversity. Overfishing, habitat degradation, and stock collapse followed.

This phenomenon is what sociologist Boaventura de Sousa Santos calls epistemicide: the systematic erasure of alternative ways of knowing. In this case, it was the loss of an entire computational system embedded in material culture and oral tradition.

Bringing it Back: The Ethnomathematical Revival

Today, researchers and fishers are working together to revive these ancestral models. In Maluku, traditional sasi closures, rotational fishing bans based on lunar cycles, have been paired with GPS tracking and drone surveillance to create ‘Sasi 2.0,’ a hybrid conservation model. In Lombok, digital tools are being developed to calculate pencar net sequences using mobile apps, preserving the formulas while adapting them to new contexts.

These efforts are often grassroots-led, supported by universities and local NGOs, and reflect a broader shift in conservation science: moving away from top-down models toward community-based co-management. They also reflect what some scholars have termed the ‘ethnocomputational revival,’ a revalidation of non-Western algorithmic traditions.

This work isn’t about nostalgia. It’s about recognising the validity of Indigenous science and restoring parity between different knowledge systems. Where colonial authorities saw superstition, we now see sophisticated ecological mathematics.

Public initiatives such as community mapping workshops, ‘net-making days,’ and storytelling circles are increasingly being used to document ancestral fishing knowledge. These formats allow elders to teach younger generations how algorithms of the past can be relevant for future conservation.

This revival is also taking shape in education. In several Indonesian schools, teachers are beginning to include ethnomathematics modules in their curriculum, bringing students into contact with the mathematical foundations of their own communities. By teaching algorithms alongside stories, these programmes reinforce the cultural embeddedness of science.

In parallel, some universities and museums are now creating digital archives of traditional fishing practices, allowing both scholars and practitioners to study the structural logic of pencar nets and bubu traps. These resources help preserve a knowledge system that colonial regimes once tried to erase, and create space for future innovations grounded in ancestral wisdom.

Even at international policy levels, some NGOs and development agencies are beginning to explore how integrating Indigenous ecological models can improve marine conservation outcomes. These include proposals for co-management frameworks that not only share governance but also incorporate traditional calculation systems into regulatory mechanisms.

Why it matters

Indigenous knowledge isn’t just cultural heritage; it’s often more sustainable and locally appropriate than imported models. As global fisheries face collapse, these practices offer pathways to resilience. By decolonising science and recognising that mathematics exists beyond Western canons, we not only honour the past but equip ourselves for a more inclusive and sustainable future.

Mathematics is often taught as a universal language. But who decides which formulas get to speak? When we elevate Indigenous algorithms like those found in pencar nets or bubu traps, we begin to tell a more complete, more truthful story of mathematics as a human enterprise.

Reclaiming these epistemologies also has a political dimension. It challenges the lingering colonial assumptions in marine policy and education systems, and affirms that sustainable development cannot be separated from cultural respect.

Whether you’re a mathematician, historian, or simply someone who loves the sea, there’s something profound about realising that a fishing net isn’t just rope, it’s an equation in action. One that has been calculated, tested, refined, and now revived.

Want to Hear More?

As part of my VIP module, I was interviewed for a forthcoming documentary produced by the Eden River VIP project. While the documentary is still in production, my segment, linked below, focuses on the parallels between traditional Indonesian fishing systems and current debates on river conservation in the UK. I reflect on how Indigenous and scientific knowledge systems can work together to challenge dominant views of sustainability and offer alternative, place-based approaches to environmental care.

Fishing as Mathematics: The Pencar Net

The Geometry of Traps: Bubu Structures